Multiple Choice
Identify the
choice that best completes the statement or answers the question.
|
|
|
1.
|
Use fundamental identities to simplify the
expression below and then determine which of the following is not equivalent.
|
|
|
2.
|
Use a graphing utility to determine which of the
trigonometric functions is equal to the following expression.
|
|
|
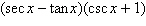
3.
|
If  , use trigonometric
substitution to write  as a trigonometric function of q, where  .
|
|
|
4.
|
Rewrite  as a single logarithm and
then simplify the result.
|
|
|
5.
|
Use the cofunction identities to evaluate the
expression below without the aid of a calculator.
a. | 0 | b. | 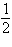 | c. | 1 | d. | –1 | e. | 2 |
|
|
|
6.
|
Solve the following equation.
|
|
|
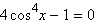
7.
|
Use a graphing utility to approximate the solutions
(to three decimal places) of the given equation in the interval  .
|
|
|
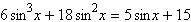
8.
|
Use inverse functions where needed to find all
solutions (if they exist) of the given equation on the interval  .
a. | 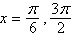 | b. | 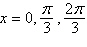 | c. | 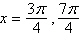 | d. | 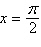 | e. | solution does not exist |
|
|
|
9.
|
Find the exact value of the given
expression.
|
|
|
10.
|
Find the exact value of the given expression using
a sum or difference formula.
|
|
|
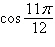
11.
|
Find the exact value of the given expression using
a sum or difference formula.
|
|
|
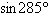
12.
|
Write the given expression as the tangent of an
angle.
|
|
|
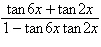
13.
|
Simplify the given expression
algebraically.
|
|
|
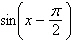
14.
|
Find the exact solutions of the given equation in
the interval  .
|
|
|
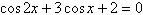
15.
|
Use a double angle formula to rewrite the given
expression.
|
|
|
16.
|
Use the half-angle formulas to determine the exact
value of the given trigonometric expression.
|
|
|
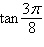
17.
|
Use the half-angle formula to simplify the given
expression.
|
|
|
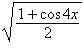
18.
|
Use the product-to-sum formula to write the given
product as a sum or difference.
|
|
|
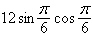
19.
|
Use the sum-to-product formulas to write the given
expression as a product.
|
|
|
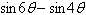
20.
|
The range of a projectile fired at an angle
q with the horizontal and with an initial velocity of  feet per second is  where r is measured in feet. A golfer
strikes a golf ball at 120 feet per second. Ignoring the effects of air resistance, at what angle
must the golfer hit the ball so that it travels 140 feet? (Round answer to nearest
angle.)
|